
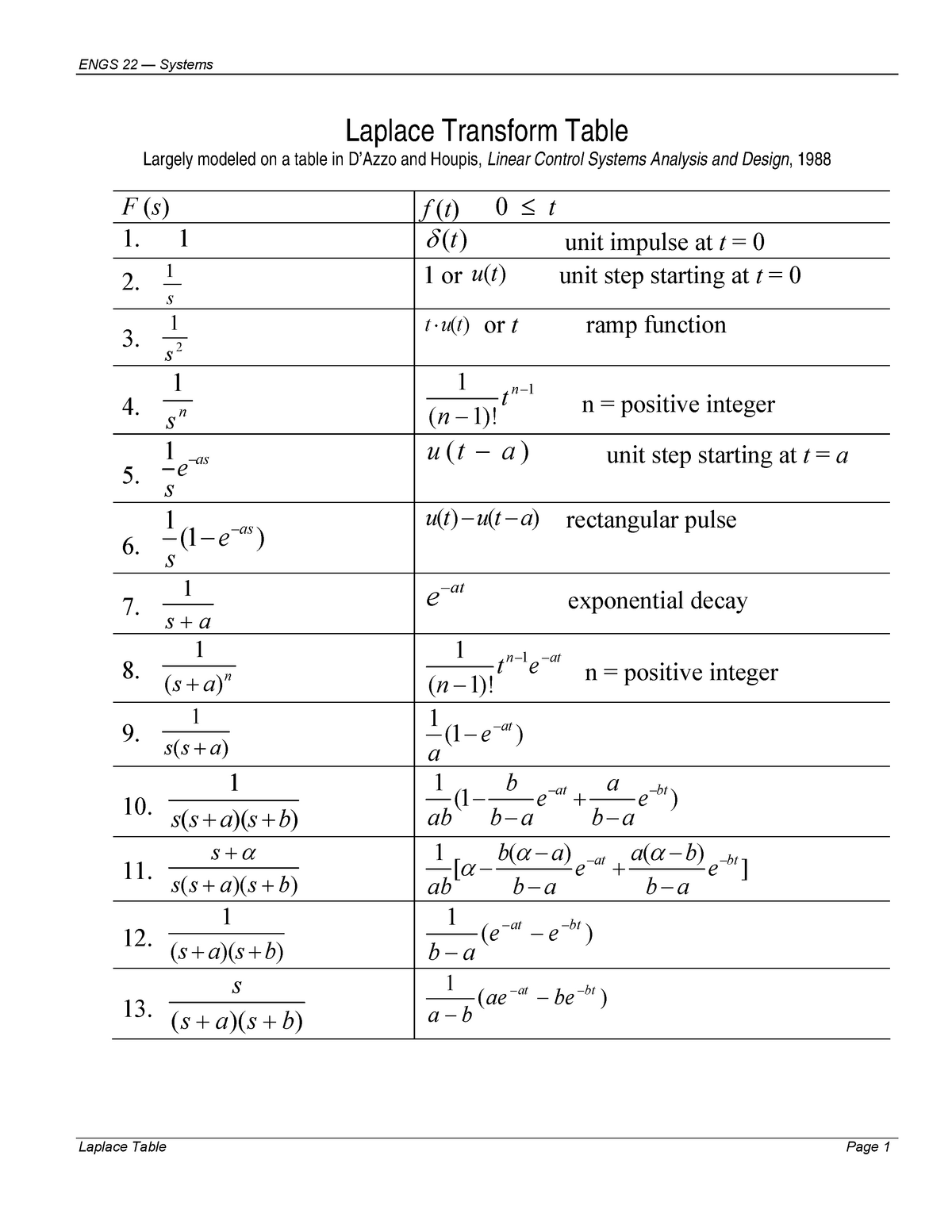
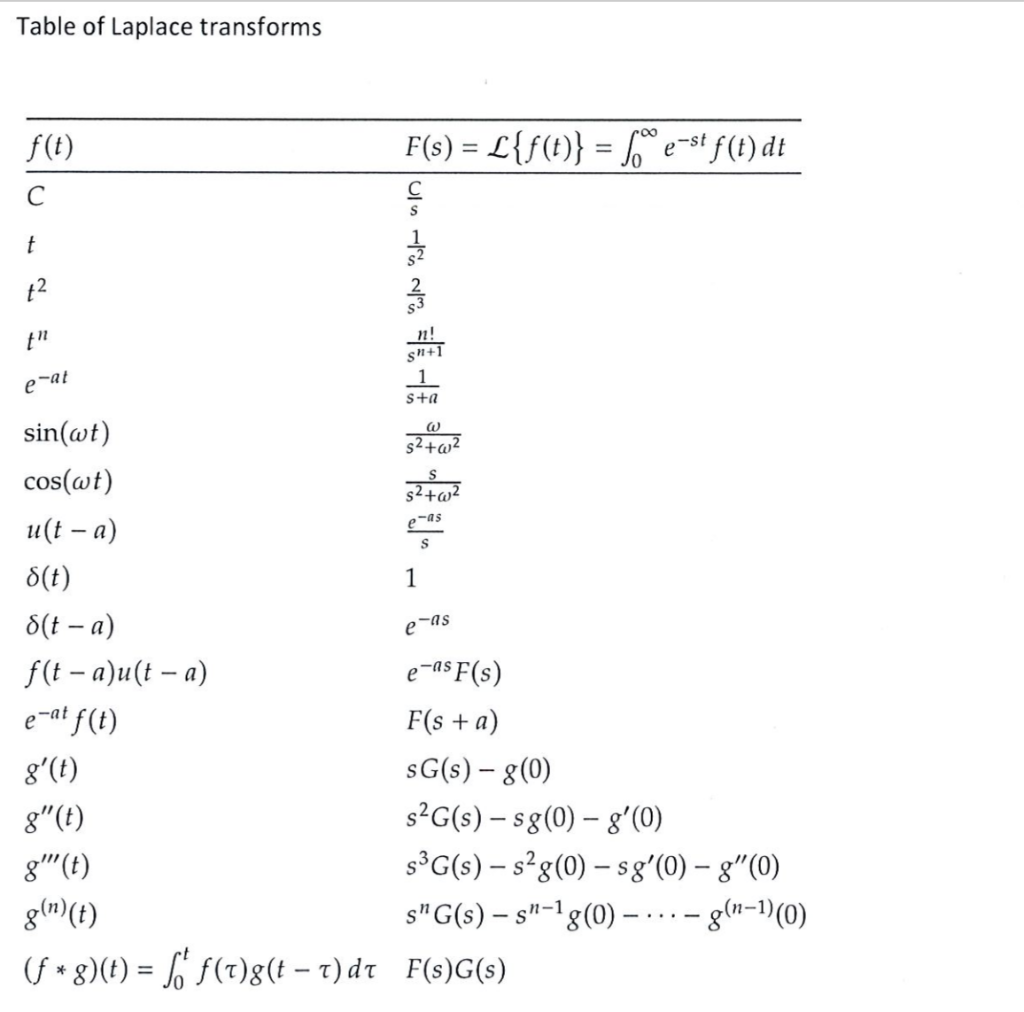
Usually we just use a table of transforms when actually computing Laplace transforms. Table 2.2.2 Properties of the Laplace transform X(s)=Jof()e dt aF(s)bG (s) x(t) af (t)bg (t) 1. This material represents a collection of integrals of the Laplace- and inverse Laplace Transform type. Laplace Transforms As we saw in the last section computing Laplace transforms directly can be fairly complicated. A: Laplace transform converts the real variable into complex variable. (s2+b2) 2hs 28 (s2h2) sin bt 2-b2 29 (s2+h2) t cos bt (cos btcos b2) (bih3) 30. Q: Q2 Find the inverse Laplace Transform of the function in figure below s² + 6s + 7 L-1 s² + 3s + 2. $2(82 +b2) sin bt bt 2b3 sin bt- bt cos bt 27.
AN INTRODUCTION TO DIFFERENTIAL EQUATIONS AND THEIR APPLICATIONS AN INTRODUCTION TO DIFFERENTIAL EQUATIONS AND THEIR APPLICATIONS STANLEY J.

(s +a)(s+b)(s + c) (b-a)(c-a) (c-b)(a -b) (a-c)(b c) (p-be + (c- b)(a - b) (p-cle + (a -c)(b-c) (p-a)e s+ p 16. TABLE OF INVERSE LAPLACE TRANSFORMS TABLE OF INVERSE LAPLACE TRANSFORMS F(s) 1. This transformation is used to convert normal differential equations into algebraic equations that may be used to solve ordinary differential problems. constant, c u,(t-D), shifted unit step 4. What is Laplace Transform Laplace transformations describe integral transformations in math that convert a real-valued function f (t) to a complex-valued function. Table 2.2.1 Table of Laplace transform pairs. For part c, do not use # 11 in Table 2.2.1. For part b, do not use # 29 in Table 2.2.1. An inverse Laplace transform table involving fractional and irrational operations is collected in Table A.2 (see 9, 35). Specify which transform pair or property is used and write in the simplest form. Click here to view the table of properties of Laplace transforms.Transcribed image text: Use the table of Laplace transforms and properties to obtain the Laplace transform of the following functions. It is considered the best way to convert. It changes one signal into another based on some fixed rules and equations. It is named after a great French mathematician, Pierre Simon De Laplace. 1 Click here to view the table of Laplace transforms. Laplace transform is an integral transform that converts a real variable function, usually time, to a function of a complex variable or complex frequency. 2- 6s + 30 2 + 6s + 18ĭetermine the inverse Laplace transform of the function below.
Click here to view the table of properties of Laplace transforms. 6s + 30 2 s' + 6s + 18 Click here to view the table of Laplace transforms. 3 3² +9ĭetermine the inverse Laplace transform of the function below. CD 3 ² + 9 Click here to view the table of Laplace transforms. 2(s)=ĭetermine the inverse Laplace transform of the function below. Transcribed image text: Use the Laplace transform table and the linearity of the Laplace transform to determine the following transform Complete parts a and b below.


 0 kommentar(er)
0 kommentar(er)
